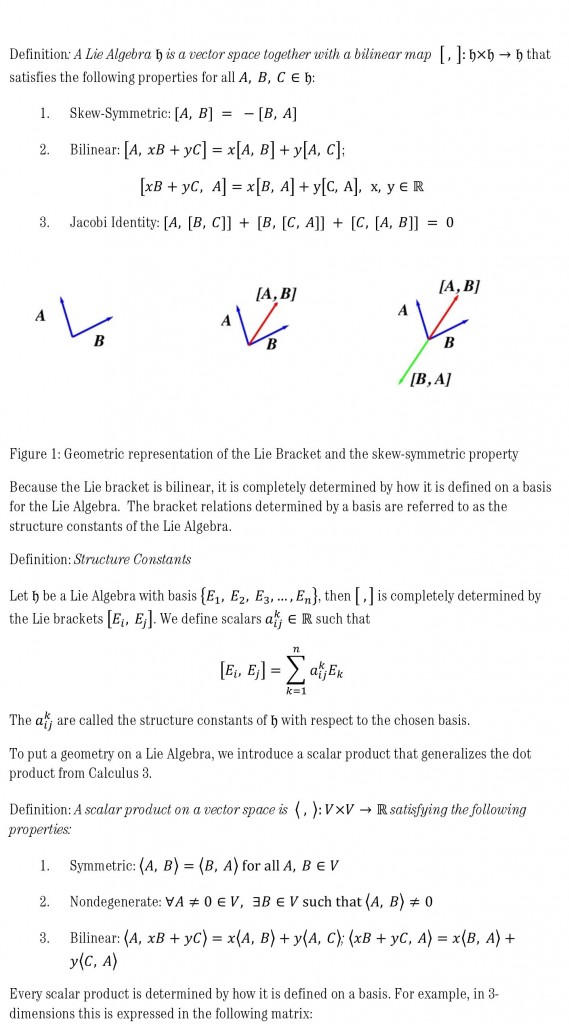
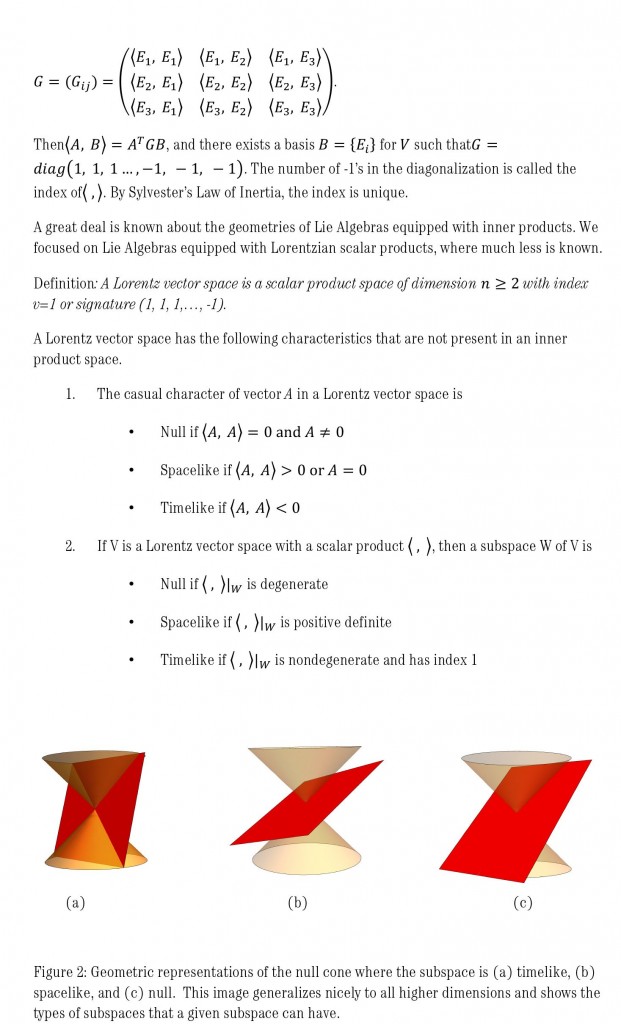
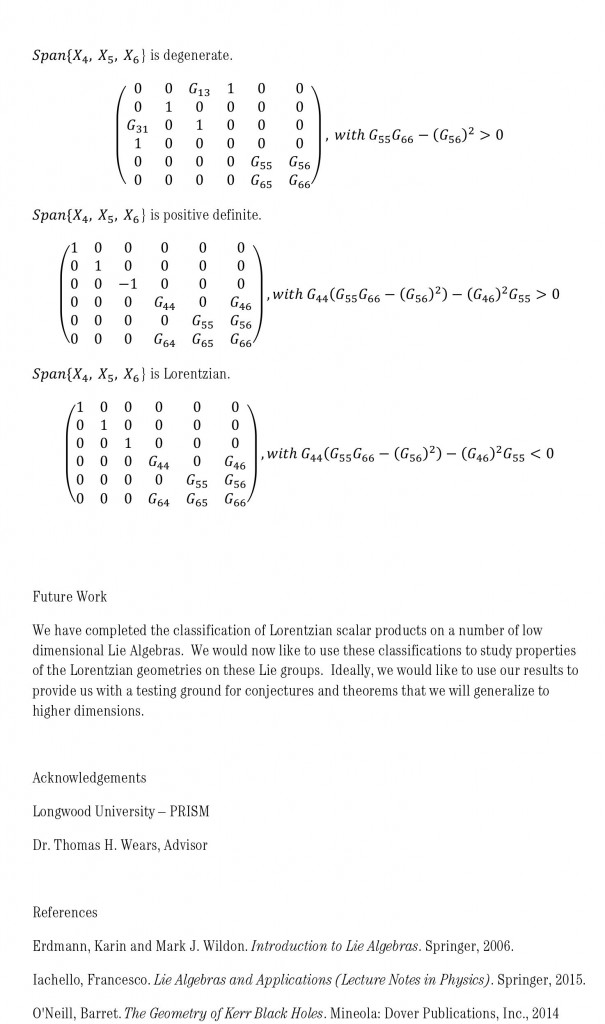
Sabrina Walker, author
Dr. Thomas Wears, faculty advisor
awarded first place in the poster catagory
Introduction
This research project is a preliminary step toward investigating the Lorentzian geometry of low-(four, five, and six) dimensional Lie Algebras. The Lorentzian geometry of a Lie Algebra is determined by both the Lie Algebra structure and the choice of a Lorentzian scalar product for the underlying vector space structure. For an n-dimensional Lie Algebra, this provides one with (n(n+1))/2 free parameters (subject to the appropriate restrictions) for the scalar product, which are far too many to be able to analyze the different Lorentzian geometric properties that a given Lie Algebra can support. However, since both the Lie Algebra structure and the scalar product are linear structures on the underlying vector space, they are determined by how they are defined on a basis fo¬¬r the vector space. This leads to the hope that by using a preferred basis for the vector space, one can reduce the number of free parameters for the scalar product. As a first step toward trying to analyze the possible Lorentzian geometries on a particular Lie Algebra, we use automorphisms of the Lie Algebra to try to find a basis that makes the scalar products as simple as possible.